Major research outcome
- KNGPDL develops a spatial interpolation method for the stochastic particle Fokker–Planck model using polynomial reconstruction
- 관리자 |
- 2024-12-30 16:23:22|
- 160
- 2024-12-30 16:23:22|
As the world is entering the new space era, accurate and efficient flow analysis has become crucial for space vehicle design. In the upper atmosphere, the Navier-Stokes equations no more valid, For this rarefied gas flow, the Direct Simulation Monte Carlo (DSMC) method based on the Boltzmann equation is widely used. Although the DSMC method applies to both rarefied and continuum flows, its computational cost grows rapidly at higher densities due to the increase in particle collisions. To address this problem, Jenny et al. proposed a stochastic particle Fokker-Planck (FP) model that replaces particle collisions with the Brownian motion. Although this linear FP model enhances computational efficiency, it fails to predict the correct Prandtl number for monatomic gases. While advanced FP models have improved physical accuracy by recovering the correct Prandtl number, their first-order accuracy in space and time limits grid and time step sizes. Recently studies have focused on enhancing the temporal accuracy of the FP model, but improving spatial accuracy remains challenging.
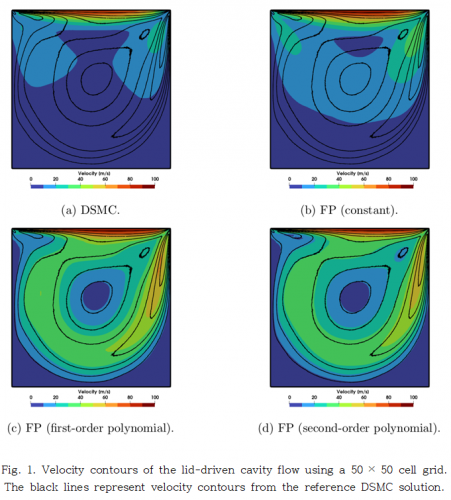
In the KAIST Non-Equilibrium Gas and Plasma Dynamics Laboratory (KNGPDL) of the Department of Aerospace Engineering, master’s student Joonbeom Kim and Professor Eunji Jun developed a spatial interpolation method based on polynomial reconstruction to improve the spatial accuracy of the FP model while preserving cell-averaged properties. Fig. 1 shows the velocity contours of the lid-driven cavity flow. The results from the FP (first-order polynomial) and FP (second-order polynomial) methods are compared with those of the DSMC method and the FP (constant) method. On a 50x50 grid, the FP methods with the spatial interpolation method shows high accuracy results.
This research has been published in Volume 36 of the Physics of Fluids (POF) journal and selected as an Editor’s Pick. Physics of Fluids is a leading journal in fluid mechanics, known for publishing high-quality research across the field of flow dynamics (IF: 4.1, top 5% in JCR).
Title : A spatial interpolation for a stochastic particle Fokker–Planck model using a polynomial reconstruction
https://doi.org/10.1063/5.0247494
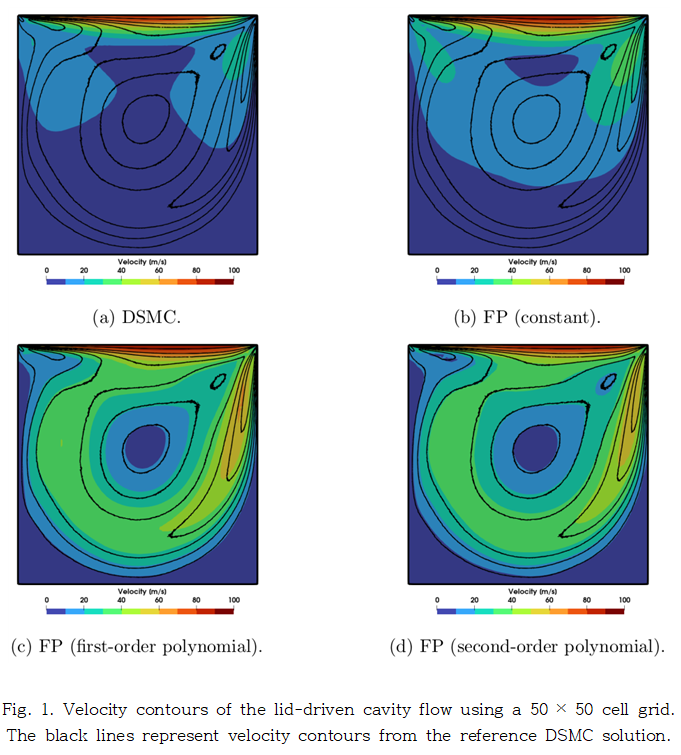
In the KAIST Non-Equilibrium Gas and Plasma Dynamics Laboratory (KNGPDL) of the Department of Aerospace Engineering, master’s student Joonbeom Kim and Professor Eunji Jun developed a spatial interpolation method based on polynomial reconstruction to improve the spatial accuracy of the FP model while preserving cell-averaged properties. Fig. 1 shows the velocity contours of the lid-driven cavity flow. The results from the FP (first-order polynomial) and FP (second-order polynomial) methods are compared with those of the DSMC method and the FP (constant) method. On a 50x50 grid, the FP methods with the spatial interpolation method shows high accuracy results.
This research has been published in Volume 36 of the Physics of Fluids (POF) journal and selected as an Editor’s Pick. Physics of Fluids is a leading journal in fluid mechanics, known for publishing high-quality research across the field of flow dynamics (IF: 4.1, top 5% in JCR).
Title : A spatial interpolation for a stochastic particle Fokker–Planck model using a polynomial reconstruction
https://doi.org/10.1063/5.0247494

| Attach File |
|---|